C++阶乘函数（递归）详解

关键词

C++阶乘函数（递归）详解

递归计算阶乘函数将接收一个整数参数并计算其阶乘。现在不妨用数学的例子来检验一个递归的应用。

在数学中，符号 n! 表示数字 n 的阶乘。整数 n 的阶乘被定义如下。

n! = 1 X 2 X 3 X … X n; 如果 n>0
n =1; 如果 n = 0

规则规定，当 n 大于 0 时，其阶乘是从 1 到 n 的所有正整数的乘积。例如，6! 可以计算为 1X2X3X4X5X6。规则还规定了基本情况：0 的阶乘是 1。

可以使用递归定义一个数的阶乘，如下所示：

factorial(n) = n X fafactorial(n-1); 如果 n> 0
factorial(n) = 1; 如果 n = 0

这个递归定义的 C++ 实现语句如下：

int factorial(int num)
{
    if (num == 0) //基本情况返回1;
        return 1;
    else
        return num * factorial(num - 1);
}

来看一个显示 3! 的值的程序，其语句如下：

cout << factorial(3) << endl;

第一次调用该函数时，num 被设置为 3，所以 if 语句将执行以下代码行：

return num * factorial(num - 1);

虽然这是一个 return 语句，但它并不会立即返回，这是因为在确定返回值之前，必须确定 factorial(num-1) 的值。该函数被递归调用，直到第 4 次调用，其中的 num 参数将被设置为 0。图 1 中直观地说明了函数每次调用期间 num 的值和返回值。

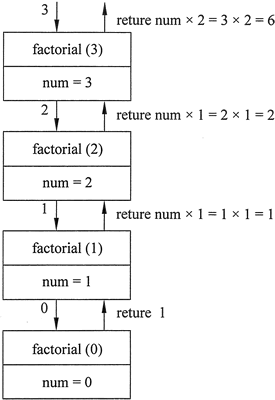
图 1 递归阶乘函数图解

下面的程序演示了 factorial 函数的使用:

// This program demonstrates a recursive function
// to calculate the factorial of a number.
#include <iostream>
using namespace std;

// Function prototype
int factorial(int);

int main()
{
    int number;
    cout << "Enter an integer value and I will display\n";
    cout << "its factorial: ";
    cin >> number;
    cout << "The factorial of " << number << " is ";
    cout << factorial (number) << endl;
    return 0;
}
int factorial(int num)
{
    if (num == 0) //base case
        return 1;
    else
        return num * factorial(num - 1);
}

程序输出结果：

Enter an integer value and I will display
its factorial: 4
The factorial of 4 is 24

本文链接：http://task.lmcjl.com/news/15929.html

展开阅读全文

上一篇：CSS多列布局下一篇：CSS过渡效果

热门文章排行

推荐文章

关键词

C++阶乘函数（递归）详解